Calculations for Wires
Jordan provided basic calculations. To further analyze professional structural information of the product refer to US cargo control.
Calculations have been provided to further the analysis of the wire
NOTICE: Calculation is an unrealistic representation of project design. Calculations are done this way just to provide evidence of sound structure requirements.
Assumptions:
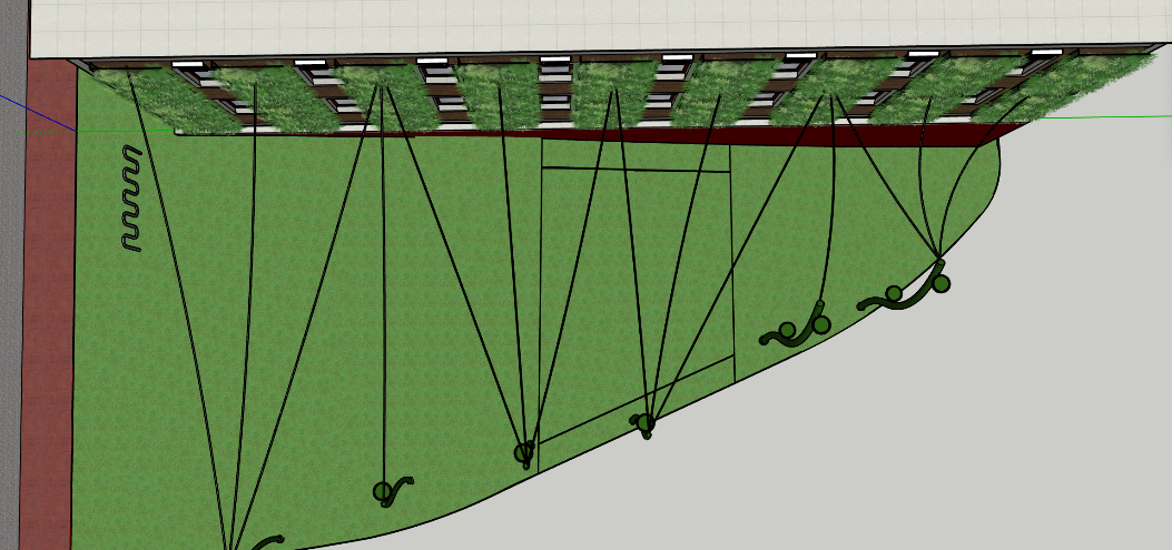
210 lbs weight directly in the middle of the structured wire.
Assumed 45-degree angle exit point for wires
Knowns:
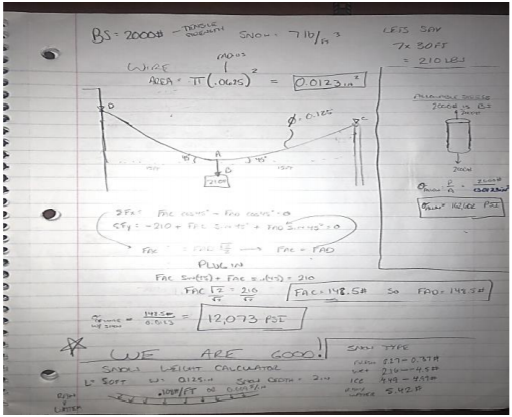
Area of wire= 0.0123sqin
Break Strength (BS)= 2000 lbs
Normal allowable stress:
Normal allowable stress= BS/A= 2000 lbs/0.0123sqin
Normal allowable stress= 162,602 PSI
Wire on each side of 210 lbs weight holds 148.5 lbs
Normal Stress on Wire= 148.5 lbs/0.0123sqin
Normal Stress on Wire= 12,073PSI
12,073 PSI < 162,602 PSI So wire is safe and will not break
Snow weight calculator- Done to provide realistic evidence on our wire
Wire width= 0.125in
Snow depth= 2 in
L= 50 ft max length of wire distance
Weights below are calculated based on
Fresh snow 0.27-0.37 lbs
Wet snow 2.16-4.5 lbs
Ice 4.49-4.99 lbs
rain/water 5.42 lbs
* No rain, snow, or water will be able to sit on ⅛ in diameter wire at 2 in
Taking the max weight of 5.42 lbs calculate the max load upon the wire as a whole for 50ft
Load=5.42 lbs/50ft
Load=.108 lbs/ft or .0009 lbs/in
Loads must be extremely heavy in one given point to cause failure of break strength. Due to the design of the project, the wire will not encounter any super heavy loads. Realistically the only loads it may experience are snow, rain, or a small animal, which will not provide enough stress to cause failure to wire rope.
Calculations for Spires
Jordan provided basic calculations. To further analyze professional structural information of the product refer to Valmont Structures.


Calculations provided further the evidence for this spire being the top choice. The calculations were completed using the estimated projected area calculations along with simple shear stress calculations. Based upon the equation engineers use to understand force from wind and the stress it places on the spire, the spire shows no results of failure.
Equation
F=A x P x Cd x Kz x Gh
A= area wind hits (Varied upon height of spire)
P=Wind pressure
Cd= Drag Coefficient
Kz= Exposure
Gh= gust factor
Wind pressure equation
P=0.00256(V)^2 where V is wind speed
V=70 mph based on American Association of State Highway and Transportation Officials
Cd Long cylinders standard coefficient
Cd=1.2
Kz equation
Kz=(z/33)^(2/7)
z= from ground to midpoint of structure
Gust factor
Gh=1.3 given from Valmont Structure website (load and dimensional data)
Wind Gusts from North and South will provide force
S0-01 16’ spire= 148.55 lbs
S0-02 20’ spire= 196.74 lbs
S0-03 25’6” spire= 268.6 lbs
S0-04 28’6” spire= 312.12 lbs
Shear strength
1100-0 is the weakest aluminum metal
Known shear strength (T) = 9,500psi
Test our structures greatest force applied to it to see if it fails
T= F/A
A= is smallest diameter of spire, most likely near top of spire
T=312.12 lbs/2in
T=156.06 PSI
Calculations for Cap of Spire with 3 wires
Jordan provided basic calculations. To further analyze professional structural information of the product refer to Valmont Structures and US Cargo Control.
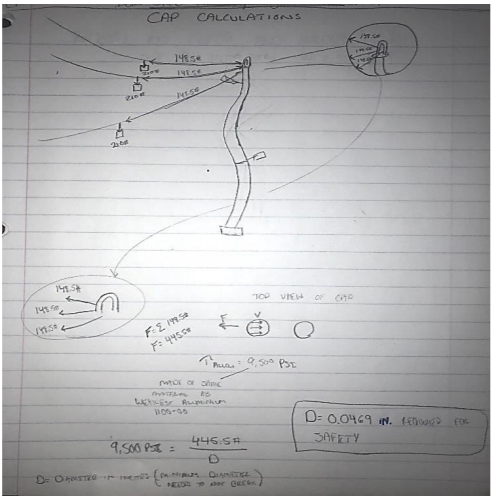
Note* Structural integrity will most likely be determined by the furnishing of spire caps.
For the spires with three wires connecting to it, they will have the highest load and force applied to the spire cap. The calculations below determine the minimum diameter of the cap connector to ensure the wires do not cause failure to the connector.
1 Wire = 148.5 lbs refer to Calculation for Wire for more information.
Cap with 3 wires
F=445.5 lbs
Shear stress allowed (T)= 9,500psi from 1100-0 Weakest Aluminum Metal
Stress Equation
9,500psi= 445.5 lbs/D
D= minimum diameter needed for safe structure
D=0.0469 inches
Structure will be structurally safe as long as cap is furnished with aluminum and has a diameter equal to or greater than 0.0469 in.
All structures should be feasible for use.